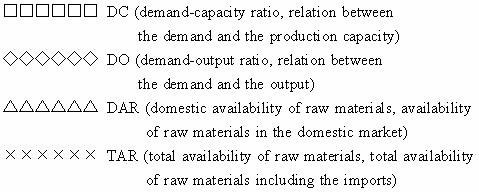
|
CONTENTS
PREFACE
Section
I. INTRODUCTION
II. THEORETICAL FRAMEWORK
1 Some Theories on Investment Decision
2 Possibility Index
3 Method of Analysis
III. MANUFACTURING INDUSTRIES IN INDIA
1 Cement Industry (3)
2 Electric Motor Industry (6)
3 Ceramic Insulator (Low Tension) Industry
(7)
4 Lead Industry (8)
IV. MANUFACTURING INDUSTRIES IN JAPAN
V. FORMULATION OF THE INVESTMENT CRITERION
1 The Way to Calculate the Possibility Index
2 Calculation of the Desired Capacity
3 Calculation of the Possibility Index
4 Formulation of the Possibility Index
VI. CONCLUSION
APPENDICES
1 Source and Note of the Figures
2 Figures
3 TABLES
NOTES
SELECTED BIBLIOGRAPHY
1 Bibliography in English
2 Bibliography in Japanese
PREFACE
The topic of this paper is, as the title
shows, to formulate an investment criterion.
The research was focused on the Indian economy.
But it is also useful for the economy of
developed countries.
I. INTRODUCTION
The topic of this paper is, as the title
shows, to formulate an investment criterion.
Author has devised an investment criterion
and named it the Possibility Index (hereafter
called the PI). This is the index which shows
the possibility of growth of each sector.
Here, the industry is classified into each
sector such as the Diesel Engine Industry,
the Cement Industry, the Lead Industry, etc.
The basic theory is to allocate the limited
resources preferentially into the sector
of which the PI is relatively high or the
possibility of growth is relatively high.
The research is focused on the Indian economy.
But the PI is applicable to other economies
also. The contents of each section are as
follows.
In section II, the theoretical framework
of PI is shown. Here, the exact way to calculate
the PI is not specified. It is specified
after examining the Indian and the Japanese
economy. And, the method of analysis of each
sector of the Indian and the Japanese economy
is shown.
In section III, the manufacturing industry
in India is analyzed according to the method
prescribed above.
In section IV, the manufacturing industry
in Japan is analyzed. From the comparison
between these two countries, some serious
problems in Indian economy were found.
In section V, the method to work out the
PI is specified taking account of the results
of survey of both economies and the PI is
worked out with regard to each sector.
In section VI, the final conclusions are
drawn.
In NOTES, notes are collected.
In the appendix, all the figures and the
tables are collected.
II. THEORETICAL FRAMEWORK
1 Some Theories on Investment Decision
In this section, some theories on investment
decision in the development economics and
the macroeconomics are briefly surveyed.
Especially, they were surveyed with regard
to the factors taken into account and the
determinant factors of in vestment decision.
J. J. Polak and N. S. Buchanan developed
the Capital-Turnover Criterion.*1 Polak concluded that resources must be allocated
to the project where the rate of turnover
is the highest.
Alfred E. Kahn criticized Polak and Buchanan
and developed the Social Marginal Productivity
(SMP) Criterion.*2 This criterion aims to maximize the national
product by allocating the resources in the
manner that makes the SMP of investment equal
among all the projects.
Hollis B. Chenery advanced this theory by
taking account of the effect of investment
on the balance of payment.*3
Walter Galenson and Harvey Leibenstein criticized
the SMP criterion and developed the reinvestment
criterion.*4 This criterion aims to make the investment
into the project from which the largest amount
of saving per unit of capital invested can
be derived.
Amartya K. Sen reviewed the capital-turnover
criterion, the SMP criterion, and the reinvestment
criterion and put forward a fourth criterion
which he called the time series criterion.*5 This criterion aims to maximize the output
within a certain period of time.
Krishna K. Singh mentions, although this
is not his original theory, the ratio of
labor to investment criterion.*6 This criterion aims to maximize the employment
per unit of additional capital. Ragnar Nurkse
supports this idea.*7
Economists like Rosenstein-Rodan, Nurkse,
etc. supported the balanced growth.*8 On the other hand, economists like Albert
O. Hirschman, Charles P. Kindleberger, Paul
Streeten, etc. supported the unbalanced growth.*9
The Cost-Benefit Analysis takes account of
the cost-benefit ratio.
In theories on external economies and economies
of scale, Tibor Scitovsky distinguished two
types of external economies.*10 One is discussed in the equilibrium theory
and another is discussed in the theory of
industrialization in underdeveloped countries.
Chenery cited this Scitovsky's example of
external economies and calculated the effect
of it following this example.*11
Larry E. Westphal showed some ways of calculating
the effect of economies of scale using the
method of MIP (Mixed Integer Programing).*12 Furthermore, Chenery and Westphal calculated
the effect of economies of scale and timing
of investment using a model with realistic
assumption about the nature of horizontal
and vertical interdependence.*13 On the other hand, Westphal and Jacques
Cremer extended the aforementioned Chenery's
analysis on the interdependence of investment
decisions by analyzing the effect of interdependence
on make-buy decisions under the assumption
of existence of economies of scale.*14
On the other hand, in the field of macroeconomics,
John M. Keynes developed the theory of marginal
efficiency of capital.*15
In the theory of acceleration principle,
the investment is dependent on the change
in output or GNP. But, Chenery introduced
factors such as the flexible accelerator,
the lag between the changes in demand and
the new investment to meet new demand, and
the optimum degree of overcapacity.*16
D. W. Jorgenson developed a new theory called
the neoclassical theory of investment.*17 In the final result namely in the investment
function, the investment depends on the desired
capital stock, and so it depends on the user
cost of capital.
Tobin's q theory forms the main stream of
recent investment theories.*18 In this theory, the adjustment cost is taken
into account. He mathematically got the following
results.
(1) q > 1 ===> Net Investment >
0
(2) q = 1 ===> Net Investment = 0
(3) q < 1 ===> Net Investment <
0.
2 Possibility Index
This is an investment criterion devised by
author and this is the topic of this
paper. The PI shows the possibility of growth
of each sector, for example, sectors such
as the diesel engine industry and the cement
industry. The PI aims to raise the efficiency
of economy as a whole by allocating the resources
preferentially into the sectors where the
PI is relatively high, namely where the possibility
of growth is relatively high. In other words,
the PI aims to find the sectors of which
the production capacity should be expanded
or cut most preferentially. The PI is worked
out by the following formula for each sector
and every year.
DCAPij
PIij = -------- (II. 1),
CAPij
i: year,
j: code number of sector,
PI: Possibility Index,
DCAP: desired production capacity,
CAP: actual production capacity.
Namely, the PI is the ratio between the desired
production capacity and the actual production
capacity. CAP is available from the existing
statistics as will be shown later. But DCAP
must be worked out. The way to work out DCAP
will be specified after examining the results
of survey on the Indian and the Japanese
manufacturing industry. But the major variables
of DCAP are as follows.
DCAPij = F(DOij, DCij, CUij, ARij) (II. 2),
DO: relation between demand and output (demand =
domestic demand + foreign demand),
DC: relation between demand and production capacity,
CU: capacity utilization,
AR: availability of raw materials.
The factors taken into account and the determinant
factors of investment in the investment theories
surveyed and the PI are shown in the TABLE
1. The differences between the PI and other
investment theories will be examined in the
conclusion.
3 Method of Analysis
The Indian and the Japanese manufacturing
industries were surveyed with regard to the
variables of DCAP in order to analyze how
these variables reflect the possibility of
growth. The survey was carried out, as we
will see later, by drawing the data into
the graphs and by comparing both of Indian
and Japanese manufacturing industries. By
analyzing all the data available and comparing
both Indian and Japanese manufacturing industries,
some serious problems of Indian manufacturing
industries were found.
III. MANUFACTURING INDUSTRIES IN INDIA
The purpose of analysis is to see how the
variables of DCAP, namely the variables of
the PI, reflect the possibility of growth.
Sixty-three sectors were selected for the
survey on the output, the production capacity,
and the capacity utilization.*19 From 63 sectors, 9 sectors were selected
as the examples. The criteria of selection
are the yearly fluctuation of capacity utilization
and the average capacity utilization. The
calculation was made during 27 years from
1951 to 1977. The average capacity utilization
is the arithmetic average, and the fluctuation
is calculated by the following formula.
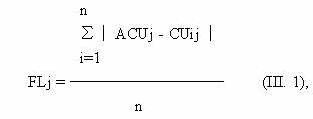
i: year
j: code number of sector,
n: number of years surveyed, in this case, 27 years,
FLj: fluctuation of capacity utilization of j sector,
ACUj: average capacity utilization of j sector during 27 years,
CUij: capacity utilization of j sector in i year.
OUT
CU = ------ X 100
CAP
OUT: output
CAP: production capacity
According to this way, following 9 sectors
were selected.
Large Fluctuation
1 Diesel Engine Industry,
2 Radio Receiver Industry,
Small Fluctuation
3 Cement Industry,
4 Soda Ash Industry,
High Capacity Utilization
5 Power Transformer Industry,
6 Electric Motor Industry,
Low Capacity Utilization
7 Ceramic Insulator Industry (low tension),
8 Lead Industry,
Others
9 Soap Industry.
The reason for selecting the Soap Industry
is that the shape of graph was interesting
to author.
The production capacity is calculated according
to the number of shift of operation. If the
shift number is 1, the production capacity
is the output produced in 8 hours operation.
If the shift number is 3, the production
capacity is the output produced in 24 hours
operation. The shift number of Cement Industry
and Soda Ash Industry is 3 and that of other
7 sectors is 1.*20
In this paper, the results of only the Cement
Industry, the Electric Motor Industry, the
Ceramic Insulator Industry, and the Lead
Industry are shown due to the restriction
on pages. The results are drawn into graphs.
For example, the graphs of the Cement Industry
are shown in the Fig. 1-5 (from Fig. 1 to
Fig. 5). The sources and notes of data are
shown in the section 1 of the Appendices.
In the graph A, the output (OUT), the production
capacity (CAP), the capacity utilization
(CU), the desired capacity (DCAP), and the
desired capacity utilization (DCU) are shown.
The unit of Y axis is percent (%). Therefore,
CU and DCU can be read directly from the
value of Y axis. But, OUT, CAP, and DCAP
are adjusted to the scale of Y axis. So the
value of them cannot be read from the Y axis.
The unit of them is shown in the note. In
case of the Cement Industry, the unit of
OUT, CAP, and DCAP is "tonnes."
The original data are OUT and CAP only. CU
was calculated by author. DCAP and DCU, which
will be mentioned in the section V, were
also calculated by author.
In the graph B, the output (OUT), the production
capacity (CAP), the exports and imports (EXPORTS
and IMPORTS), and the estimated demand (ED)
are shown in real terms. The unit of each
item is common, so each line can be compared
directly.
In the graph C, the output (OUT), the production
capacity (CAP), the output in the unorganized
sector (OUT-U), the production capacity in
the unorganized sector (CAP-U), the target
of output (O-TARGET), and the target of production
capacity (C- TARGET) are shown. The unit
is common among all items.
In the graph D, the exports (EXPORTS) and
imports (IMPORTS) in money term are shown.
This is also to see the situation of demand
and supply. The exports and imports in real
term are shown in the graph B. But the unit
of them is not necessarily appropriate. For
example, the unit of real term of the Electric
Motor Industry is "horsepower."
But there are some types of electric motors.
Namely, they are different in the horsepower
and structure. So, the figures in terms of
money is also useful. And the data of each
term are not available sufficiently, so two
types of data can compliment with each other.
In the graph E, the relation between the
demand and the output (DO), the relation
between the demand and the production capacity
(DC), the availability of raw materials within
the country (DAR, Domestic Availability of
Raw Materials), and the availability of raw
materials including the imports (TAR, Total
Availability of Raw Materials) are shown.
The meaning of each item is as follows.
The relation between the demand and the output
(DO) is divided into 11 classes.
5: The demand exceeds the output to the maximum
extent,
4: Intermediate situation between 5 and 3,
3: The supply is very inadequate,
2: The shortage of supply is clearly reported,
1: The shortage of supply is suggested,
0: The demand and the supply are equal,
-1: The excess production is suggested,
-2: The excess production is clearly reported,
-3: The production is very excessive,
-4: Intermediate situation between -3 and
-5,
-5: The production is excessive to the maximum
extent.
The relation between the demand and the production
capacity (DC) is also divided into 11 classes.
The domestic availability of raw materials
(DAR) is divided into 6 classes.
0: The raw materials are available sufficiently,
-1: The shortage is suggested,
-2: The shortage is clearly reported,
-3: The raw materials are very inadequate,
-4: Intermediate situation between -3 and
-5,
-5: The raw materials are inadequate to the
maximum extent.
The availability of raw material including
the imports (TAR) is also divided into 6
classes.
The above four items are determined subjectively,
not determined according to the objective
data. For example, if there is a report that
the demand and the output is equal, DO is
0. And if there is a report that the output
is very inadequate as compared with the demand,
DO is 3. Here, the availability of raw material
includes the availability of electric power,
transport facility, etc.
The profitability was analyzed with regard
to following 11 sectors.*21
1 Flour Mills
2 Soaps and Glycerine
3 Cement (Hydraulic)
4 Insulators
5 Typewriters and Duplicators
6 Non-Ferrous Basic Metal Industries (Including
Alloys and Their Products)
7 Internal Combustion Engines
8 Electrical Lamps
9 Radio Receivers, Including Amplifying and
Public Address Equipment
10 Electric Motors
11 Equipment for Generation, Transmission
and Distribution of Electricity Including
Transformers
Among these 11 sectors, the Cement Industry
and the Electric Motor Industry are same
as 2 sectors in 9 sectors above.
Here, the profitability is the figure calculated
from the output, the input, the fixed capital,
and the number of workers. The way of calculation
of profitability is shown by abbreviation
above each graph. The meaning of each abbreviated
letter is as follows.
I: input,
O: output,
FC: fixed capital,
M: number of workers.
For example, (O - I) / (FC + I) means the
expression
(output - input)
-------------------------------- (III. 2),
(fixed capital + input)
In this way, (O - I) / (FC + I), (O - I)
/ FC, O / FC, (O - I) / M, (O - I - FC) /
M, and FC / M were calculated and drawn on
the graphs. The result of (O - I) / (FC +
I) is shown in the Fig. 14-15. There, 11
sectors are divided into two graphs, A and
B. Other results are omitted. As written
above, these 11 sectors are different from
9 sectors for which the graphs A-E were drawn.
But Cement and Electric Motor are common
for 9 sectors and 11 sectors. The code number
of Cement is 3 and that of Electric Motor
is 10.
With regard to these data, the relative values
among 11 sectors were calculated. They were
calculated for each year by the following
formula.
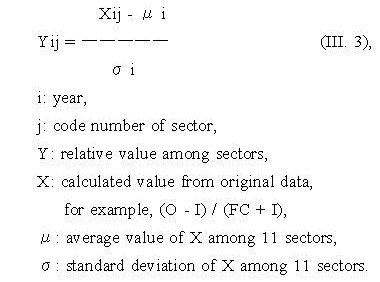
Needless to say, if Y is greater than 0 it
is higher than the average, equal to 0 the
average, and smaller than 0 lower than the
average respectively. The results are shown
only for the Cement Industry and the Electric
Motor Industry in the Fig. 16-17.
1 Cement Industry (3)*22
As written above, the code number is 3 both
among 9 sectors and 11 sectors. This sector
was selected as an example of the small fluctuation
of capacity utilization. The fluctuation
of capacity utilization is the smallest among
63 sectors. The average capacity utilization
is 86.4%. But, as mentioned earlier, the
production capacity of the Cement Industry
and the Soda Ash Industry was calculated
on the 3 shift basis. So, the 86.4% is close
to the upper limit of capacity utilization.
The graph A to E are shown in the Fig. 1-5.
In case of the Cement Industry and the Electric
Motor Industry, as mentioned above, the data
of profitability are available. The profitability
was analyzed about 11 sectors including the
Cement Industry and the Electric Motor Industry.
The profitability, (O - I) / (FC + I), of
11 sectors selected are shown in the Fig.
14-15. The relative value was worked out
by the formula (III. 3) and the results of
the Cement Industry are shown in the Fig.
16.
It can be seen in the Fig. 1 or the TABLE
2 that the output has been growing steadily
and the production capacity has been expanded
very smoothly following the output, and so
the capacity utilization has been stabilized
very well. This forms a strong contrast to
the Diesel Engine Industry and the Radio
Receiver Industry although they were omitted.
The situation of the Cement Industry looks
very well. But, some serious problems were
found in the survey.
The price and the distribution of cement
has been under control for mostly all the
period surveyed.*23 The relation that price control ===> low
price ===> small profit ===> inadequate
investment ===> shortage of cement was
often reported.*24 And the power cut, the voltage fluctuation,
the inadequate and irregular coal supply,
the shortage of wagons for the movement of
raw materials as well as cement, and the
labor troubles were the principal causes
of the low capacity utilization.*25 Sometimes the black market, where the price
was three times the official price, had emerged.*26
The control of price and distribution was
removed in order to stimulate the expansion
of industry in January 1966. So, the price
rose and, stimulated by this, the producers
ventured on the expansion of undertakings
one after another.*27 But, thereafter, the control was imposed
again and the price was fixed.*28
These situation can be observed in the
graphs. In the Fig. 2, the estimated demand
sometimes exceeds the output. In the Fig.
5, the demand-output relation (DO) often
shows the supply shortage. The domestic availability
of raw materials (DAR) are often -3, meaning
that the raw materials were often very inadequate.
On the other hand, the profitability is seen
in the Fig. 14 where the code number of Cement
is 3. The profitability did not fluctuate
largely. The relative value of profitability
among 11 sectors are seen in the Fig. 16.
There, the fixed capital per labor (FC/M)
is high but the labor productivity ((O -
I) / M, (O - I - FC) / M) is low because
the profitability of capital ((O - I) / (FC
+ I), (O - I) / FC, O / FC) is low.
Thus, the administered price and distribution
are the serious problems in the Cement Industry.
2 Electric Motor Industry (6)
As written above, the code number is 6 among
9 sectors and 10 among 11 sectors. This sector
was selected because of its high capacity
utilization. The average capacity utilization
is 106.1%, 3rd among 63 sectors and the fluctuation
of capacity utilization is 7th.
In the Fig. 6-8, in the years the supply
was inadequate, the capacity utilization
was high and the imports was much greater
than the exports.
On the other hand, in the Fig. 15 where the
code number of Electric Motor is 10, the
profitability is high in the period 1959-66
while they are not so far from the average
in the period 1968-71. In the Fig. 17, the
profitability of capital [(O - I) / (FC +
I), (O - I) / FC, O / FC] is relatively high
in the period 1959-1966 as compared to the
period 1968-1971. In the former period, larger
shortage of supply was reported as compared
with latter period (Fig. 8). The fixed capital
per worker (FC / M) is relatively low but
the profitability of capital is high and
the productivity of labor [(O - I) / M, (O
- I - FC) / M] is mostly average or higher.
3 Ceramic Insulator (Low Tension) Industry
(7)
This sector was selected as an example of
the sectors of low capacity utilization.
The average capacity utilization was 36.5%,
the lowest among 63 sectors and the fluctuation
of capacity utilization was 35th.
In the Fig. 9 and the TABLE 2, the highest
capacity utilization was 70.4% recorded in
1955 and the lowest one was 7.7% recorded
in 1972.
In the Fig. 10, from 1951 to 1955, inadequate
supply of insulators and low availability
of raw materials were reported. But in the
later period, the capacity utilization declined.
The literature concerned are not abound,
so the cause is not known exactly. But it
seems that the shortage of demand made the
capacity utilization decline. The production
capacity was not cut despite the low capacity
utilization. The reason seems to be that
this industry was protected as a small-scale
industry.*29
4 Lead Industry (8)
Also this sector was selected as an example
of low capacity utilization. The average
capacity utilization is 49.2%, 59th among
63 sectors and the fluctuation of capacity
utilization is 27th.
In the Fig. 11, the production capacity had
not shown a large change until around 1976.
The capacity utilization had stayed at low
level for mostly all the period surveyed.
From 1947 to 1950, for which the data is
not drawn into the graph, the capacity utilization
had been between 3.2% and 10.5%.*30 Therefore, for 27 years ranging from 1947
to 1973, the capacity utilization was between
3.2% in 1947 and 64.9% in 1959.
In the Fig. 12, the estimated demand shows
the much higher value than the output, and
in many years the amount of import is several
times or more than ten times the domestic
production.
In the Fig. 13, the shortage of raw materials
and the lead are often reported. The extent
of shortage is, for the raw materials from
2 to 5, and for the supply of lead from 3
to 5 respectively. This means that the shortages
are extremely serious for both the raw materials
and the supply of lead. Thus, the capacity
utilization was very low due to the inadequate
supply of lead ore and the supply of lead
was inadequate. And so the lead has been
imported by the amount several times or more
than ten times the domestic production.
We have seen that the capacity utilization
was low due to the lack of demand in the
Ceramic Insulator Industry and due to the
shortage of raw materials in the Lead Industry
respectively. As represented by these examples,
in India, the major causes of low capacity
utilization are two. One is the lack of demand
and the other is the shortage of raw materials.
Koti had conducted a survey on 517 items.
According to him, in the sectors of low capacity
utilization, 42% of them is due to the lack
of demand, 21% to the shortage of raw materials,
4% to both causes, 17% to the lack of demand,
the shortage of raw materials, the shortage
of power, the labor trouble, and the lack
of fund. Namely, 82% is related to the lack
of demand or the shortage of raw materials.*31
IV. MANUFACTURING INDUSTRIES IN JAPAN
Regarding Japan also, same type of analysis
was carried out as far as possible. Thirty
sectors were selected for the survey of the
output, the production capacity, and the
capacity utilization.*32 They were selected considering the continuity
of data. Regarding the profitability, the
data are available for 12 sectors.*33
In case of Japan, the output and the capacity
utilization are shown by the index. And the
production capacity was calculated from above
two variables by author. So the output and
the production capacity cannot be compared
directly on the graph like the Indian manufacturing
industries. And the capacity utilization
of different sectors cannot be compared directly.
But, as we will see it, relative trend of
progress can be seen from the graphs. Also
the output and the capacity utilization are
available for the above 12 sectors of which
the data of profitability are available.
Regarding the above 12 sectors, analyzed
data on the profitability are, for example,
as follows.
Current Profit
PR = ----------------,
Total Capital
PR: Profit Rate (current profit rate of total capital),
Current Profit: sales amount + non-operating income
- cost of sales - selling cost - general
administrative cost - non-operating expenses,
Total Capital: current assets + fixed assets + deferred assets.
Relative value of each data was calculated
according to the expression (III. 3).
Like the case of India, some sectors were
selected for the further survey. But the
capacity utilization is shown by the index
and the fluctuation of capacity utilization
is not so different among sectors. So the
criterion of selection is different from
that of India. Here, with regard to the adjustment
of production capacity, only the Electric
Machine Industry, the Precision Machine Industry,
the Chemical Fertilizer Industry, and the
Silk Reeling Industry are examined (Fig.
18-21). Former two sectors are the examples
of increasing rate of growth, and latter
two sectors are the examples of decreasing
output. In case of the Electric Machine Industry
and the Precision Machine Industry, the output
has been increasing with progressively increasing
rate of growth. The production capacity has
been expanded according to the increase in
the output.
On the other hand, in case of the Chemical
Fertilizer Industry and the Silk Reeling
Industry, the output has been decreasing
and the production capacity has been cut
according to the decrease in the output.
In case of the Chemical Fertilizer Industry,
major causes of the decrease in output were
two oil shocks. Also the decrease in the
demand, the overcompetition, and the loss
of international competitive power had made
this industry a structural slump ridden industry.*34 In the Fig. 22, the export of chemical fertilizer
had been increasing until 1972 and thereafter
began to decrease. It is observed that the
production capacity has been cut according
to the decrease in the exports (Fig. 20).
In case of the Silk Reeling Industry, the
output had been gradually increasing until
1969 and thereafter turned to the decreasing
trend. The silk had been the most important
goods for export until 1920's. But thereafter
the exports decreased drastically due to
the depression and the development of synthetic
fiber in the USA. Since 1966, Japan has been
importing the silk.*35 We can see that the maximum point of production
is very close to the point where the export
of raw silk ended and the import began (Fig.
23). Thus the production of the Silk Reeling
Industry has decreased due to the decrease
in demand and so the production capacity
has been adjusted according to the situation.
Regarding the profitability, the positive
relation between the growth rate of output
and the current profit rate of total capital
was observed although their graphs are omitted
here.
V. FORMULATION OF THE INVESTMENT CRITERION
1 The Way to Calculate the Possibility Index
As mentioned in section II, the Possibility
Index (PI), is the ratio between the desired
capacity and the actual capacity. It is written
again. It is calculated by the following
formula.
DCAP
PI = ------ x 100 (V. 1),
CAP
DCAP: desired capacity,
CAP: actual capacity.
CAP is available from the existing statistics,
so the surveys on the Indian and the Japanese
manufacturing industries have been conducted
in order to find the way to calculate DCAP.
Examining the data and information available,
the possible and best way to calculate the
PI seems to be as follows. DCAP is worked
out on the basis of Optimal Capacity Utilization
(OCU). The Optimal Capacity Utilization is
the rate at which the profit rate is the
maximum or the production is the most profitable.
So, it is different from a company to a company,
or from a sector to a sector. Here, OCU is
set at 90.0%. But, even if it is set at 80.0%,
it does not affect the results considerably
as will be shown later. The method of calculation
is different according to the situation of
capacity utilization, demand and supply,
availability of raw materials, etc. The method
is specified as follows according to the
situation.

In case of A, the Electric Motor Industry
can be cited as an example. In that industry,
there are many years in which the capacity
utilization was above 90.0% and the demand
was excessive as compared with the production
capacity. So the production capacity must
be expanded in order to lower the capacity
utilization to 90.0%. So DCAP is worked out
by the following formula,
OUT
DCAP = ------- (V. 2),
0.900
OUT: actual output.
Namely, DCAP is the production capacity which
yields OCU.
In case of B, the Ceramic Insulator Industry
can be cited as an example. In this case,
the capacity utilization was low due to the
lack of demand so the production capacity
should be cut according to the demand. So
in this case also, DCAP is worked out by
the formula (V. 2).
In case of C, the Lead Industry can be cited.
In case of the Lead Industry, the demand
was much larger than the production capacity
but the capacity utilization was very low
due to the shortage of raw materials. So
it is fruitless to expand the production
capacity because the company cannot increase
the out put with the increased capacity due
to the shortage of raw materials. And also,
it is fruitless to cut the production capacity
to raise the capacity utilization because
there exists a sufficient demand as compared
with the production capacity. Therefore,
it is desirable to maintain the present production
capacity and to supply sufficient raw materials
immediately. Therefore, DCAP is worked out
by the following formula,
DCAP = CAP (V. 3).
If we got DCAP by the above way, we can calculate
the desired capacity utilization (DCU). DCU
is worked out by the following formula.
OUT
DCU = ------ (V. 4).
DCAP
DCU is the rate at which the sector operates
with actual output OUT and DCAP. So OCU is
90.0% but DCU can be below 90.0% as we will
see it later.
DCAP, DCU, and the PI were worked out with
regard to the Indian 9 sectors selected according
to the way above.
2 Calculation of the Desired Capacity
For example, the situation of the Cement
Industry are shown in the Fig. 1-5. In 1951,
the capacity utilization was 89.8% and there
is no report that the supply was inadequate.
But, taking account of the situation around
this period, it seems that the supply was
inadequate (Fig. 5). So DCAP was worked out
by the formula (V. 3). From 1952 to 1955,
in 1960, and from 1963 to 1965, the capacity
utilization was at or above 90.0%, so DCAP
was worked out by the formula (V. 2). DCAP
of remaining years, except for 1958, 1959,
1967, and 1968, were worked out by the formula
(V. 3), because the capacity utilization
was below 90.0% but the supply seemed to
be inadequate. In 1958, 1959, 1967, and 1968,
the capacity utilization was below 90.0%
and the supply was adequate or excessive
(Fig. 5). So DCAP was worked out by the formula
(V. 2).
The results are shown in the Fig. 1. With
regard to DCU, it is sometimes equal to and
different from actual capacity utilization.
This depends on the situation of market as
written above. DCAP of other 9 sectors were
worked out by the similar way.
3 Calculation of the Possibility Index
We have worked out DCAP, so now we can work
out the PI by the formula (V. 1). The results
are shown in the Fig. 24-25 and the TABLE
3. Nine sectors are divided into two graphs.
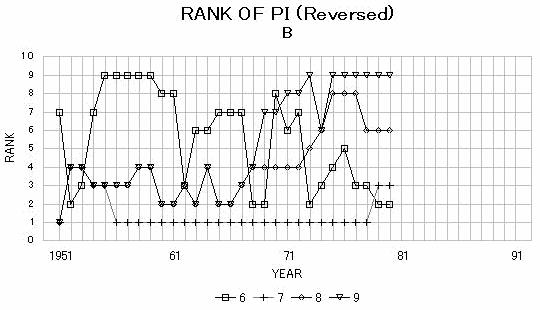
The rank of PI is shown in the Fig. 26-27.
There, the rank is reversed so that we can
optically compare the graphs of the PI and
the rank. Namely, if the PI is highest the
rank is 9, and if the PI is lowest the rank
is 1.
For example, in case of the Cement Industry
(code number of sector is 3), the PI is near
or equal to 100.0. But the rank ranges from
9th to 1st. For example, the PI is 100.0
in 1951 and 1956. But the rank is 1st in
1951 and 3rd in 1956. This is because the
rank is the relative value among 9 sectors.
Thus we can see the PI and the rank of it.
Observing all the 9 sectors, we can see following
situation. In the first decade namely from
1951 to 1960, the PI of the Electric Motor
Industry (6) shows high value, and the Ceramic
Insulator Industry (7) shows low value. Their
ranks are at the top or the bottom in some
years respectively. From 1961 to 1970, the
Diesel Engine Industry (1) and the Power
Transformer Industry (5) show high value,
and the Ceramic Insulator Industry (7) shows
low value. The ranks of Diesel Engine Industry
(1) and the Power Transformer Industry (5)
are at the top in some years, and the rank
of the Ceramic Insulator Industry (7) is
always at the bottom. From 1971 to 1980,
the Power Transformer Industry (5) and the
Soap Industry (9) show high value, the Diesel
Engine Industry (1) and the Electric Motor
Industry (6) declined to low level, and the
Ceramic Insulator Industry (7) turned to
the rising trend after showing the extremely
low level. The Power Transformer Industry
(5) and the Soap Industry (9) are at the
top rank in some years respectively. The
Diesel Engine Industry (1) declined to the
bottom rank, and the Electric Motor Industry
(6) declined to the 2nd rank. The Ceramic
Insulator Industry (7) rose up to the 3rd
rank after staying at the bottom for 23 years.
As for the Lead Industry (8), although the
capacity utilization was very low in many
years, the PI is 100.0 in most years due
to the reasons mentioned above.
4 Formulation of the Possibility Index
So far, we have examined the data available
and have worked out the PI from them. So,
we must specify the necessary and technically
possible data for the PI and the way to work
out the PI with them.
The calculation of the PI is carried out
according to the formula (V. 1). The basis
of calculation is OCU (optimal capacity utilization).
OCU is the rate at which the profit rate
is the maximum. In the calculation above,
OCU was set at 90.0%. But even if it was
set at 80.0% or at other values, the results
does not affect seriously at least with regard
to the sectors near the top rank or the bottom
rank. We have seen it above. So far, we have
adopted 90.0% as OCU for all the sectors
concerned. But we should specify OCU for
each sector. For example, in the iron and
steel industry, the blast furnace cannot
be turned off so easily, so the operation
must be continued throughout the day. Therefore,
OCU must be necessarily close to 300.0% on
the one shift basis. But, in many industries,
OCU can be far below 300.0%. In case of cement
industry, the capacity utilization was shown
on three sift basis but in other industries
the capacity utilization was shown on one
sift basis. Also this problem must be solved.
OCU must be worked out for each sector taking
account of the features of each sector. The
way to specify OCU cannot be specified here.
Author must leave this problem for the
later research.
Here, OCU was defined as the most profitable
capacity utilization. But, the capacity utilization
at which the profit rate is zero may be more
appropriate. Let us call this the minimum
required capacity utilization (MRCU). The
profit is positive above MRCU, the profit
is zero at MRCU , and the profit is negative
below MRCU. MRCU may be more easily worked
out than OCU.
From the above analysis, we can specify the
statistics necessary for the calculation
of DCAP and OCU or MRCU.
1 Output and Production Capacity,
2 Estimated Demand (including the information
on demand and supply situation of the products
in the market),
3 Availability of Raw Materials (including
the electric power, transport facility, labor
force, etc.),
4 Profitability,
5 Exports and Imports.
The specification of these statistics must
follow the criteria below.
1. The classification of sector must be common
among the statistics. For example, the classifications
of sector for the profitability and for the
output and production capacity are not common.
This is the serious obstacle to the analysis.
Secondly, the unit of output and production
capacity must be coordinated. For example,
in the Electric Motor Industry, the unit
is horsepower. But there are so many types
of electric motors. Some motors have small
horsepower and some have big horsepower,
and the structure of electric motor is not
same. We can solve this problem by setting
a standard product. For example, we can set
the electric motor of one horsepower and
of popular structure as a standard product.
Other types of electric motors can be converted
into this standard product in terms of the
cost of production. Namely, if the cost of
electric motor of other type is three times
the standard electric motor, one unit of
this motor is converted into three units
of the standard motor. Thus, every type of
electric motor can be converted into the
standard electric motor.
2. The classification of sector must be modified.
Namely, if some types of electric motors
can be manufactured by the same production
facility, they should be classified as in
the same sector. But, if they cannot, they
should be classified as in the different
sectors. But, for example, even if the electric
motor and the power transformers can be manufactured
by the same production facility, they cannot
be classified as in the same sector because
the purposes are different.
So far, we have worked out the PI from the
existing data. Now, we can specify the way
to work out the PI with the data specified
above. The capacity utilization adopted as
the base of calculation is OCU or MRCU. Here,
the capacity utilization is shown on one
shift basis and the capacity utilization
adopted as the basis is OCU set at 90.0%.
The way to work out DCAP is shown according
to each case bellow. Basically, the ways
of calculation are composed of three cases.

In case of A, basically, DCAP is worked out
by the following formula.
OUT
DCAP = ------- (V. 2),
0.900
OUT: output.
This is because 90.0% = or < CU and so
production capacity should be increased according
to the output. But, the case A is further
divided into four cases according to the
relation between the supply of and the demand
for product, and to the situation of availability
of raw materials.

In case A-1, the production capacity is fully
utilized but the supply is inadequate. In
this case, actual demand is greater than
the output. So, DCAP is worked out by the
formula
ED
DCAP = -------- (V. 5),
0.900
ED: estimated demand
In case A-2, further raw materials are not
available, so it is impossible to increase
the output. So, DCAP should be worked out
by the formula (V. 2).
In case A-3, the supply is adequate, so the
output needs not be increased. So, DCAP is
worked out by the formula (V. 2).
The case A-4 is divided into two cases. In
case A-4-1, the supply is adequate. In this
case, DCAP is worked out by the formula (V.
2) because the output needs not be increased.
In case A-4-2, the supply is inadequate and
the raw materials are inadequate. If the
raw materials are available sufficiently,
the output will increase. In this case, the
output needs to be increased in order to
meet the demand. But it is impossible to
increase the output because the raw materials
are inadequate. So, DCAP is worked out by
the formula (V. 2), not by the formula (V.
5). Thus in case A as a whole, only in case
A-1, DCAP is worked out by the formula (V.
5). In other cases, DCAP is worked out by
the formula (V. 2).
In case B, DCAP is worked out by the formula
(V. 2). Because CU < 90.0% due to the
lack of demand.
In case C, this case is divided into two
cases. In case C-1,
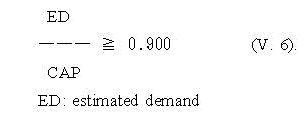
This means CAP is optimal or less as compared
with ED. So if the raw materials are supplied
sufficiently, CU will be = or > 90.0%.
But it is fruitless to increase the production
capacity because of shortage of raw materials.
So DCAP is worked out by the formula
DCAP = CAP (V. 3).
In case C-2,
ED
------ < 0.900 (V. 7).
CAP
This means CAP is excessive as compared with
ED. So DCAP is worked out by the formula
(V. 5).
As a whole, the formula (V. 2) is used in
case, A-2, A-3, A-4, and B. The formula (V.
3) is used in case C-1. The formula (V. 5)
is used in case A-1 and C-2.
Thus, we can work out DCAP more precisely
with the modified statistical system specified
above. And, the PI is worked out by the formula
DCAP
PI = ------- x 100 (V. 1).
CAP
VI. CONCLUSION
As mentioned earlier, the purpose of research
is to formulate a practical investment criterion,
not an abstract one. In this regard, it seems
that the PI is superior to other theories
in the recognition of reality. For each theory
surveyed, the factors taken into account
and the determinant factor of investment
are listed in the TABLE 1.
As a whole, the theories surveyed take account
of the profitability of firms or projects
concerned but do not take account of the
present market situation. But, as we have
seen in the analysis of the Indian manufacturing
industries, the supply of products and the
demand for it do not necessarily coincide,
and the raw materials are not always available
sufficiently. On the other hand, the PI can
incorporate these factors into its system.
This difference is quite crucial when we
need the practical investment criterion.
However, the linkage effect, which was taken
into account in the theories of the balanced
growth and the unbalanced growth and in the
theory on external economies and economies
of scale, cannot be incorporated. This is
the weak point of the PI. But, it is very
difficult to measure the linkage effect covering
the economy as a whole because the Input-Output
Table is necessary to calculate the linkage
effect over the economy as a whole. In this
case, if the industry is classified into
the sector like the data for capacity utilization,
the number of sectors will amount to several
hundreds. Furthermore, the I-O table is necessary
for every year. But, at the present situation
of statistical system, it is impossible to
work out such an I-O table every year. Furthermore,
in case of India, the data are available
from the organized sector only, not available
from the unorganized sector. So, it is mostly
impossible to work out the linkage effect
accurately. So the best way was to give up
calculating the linkage effect and to work
out the PI which does not take account of
the linkage effect.
As the conclusion, we must evaluate the reliability
of the PI as an investment criterion. The
PI, like other investment criteria, cannot
be the perfect criterion. But what we can
expect from the PI is the more efficient
investment, not the perfect investment. If,
for example, 500 sectors are selected for
the analysis and the PI of each sector is
calculated, we cannot say that the PI is
reliable with regard to two sectors of which
the ranks are 250th and 251st. But, with
regard to two sectors of which the ranks
are 1st and 250th, the PI can be highly reliable.
We can find the sectors at or near the top
rank and the sectors at or near the bottom
rank respectively. Namely, we can find the
sectors of which the production capacity
should be increased or cut most preferentially.
The immediate adjustment of the production
capacity according to the situation is
crucial for the Indian economy to grow faster,
as we have seen in the comparable analysis
on the Indian and the Japanese economy.
However, prompt adjustment of production
capacity according to the PI is not always
appropriate. For example, the military forces,
educational system, health and welfare organizations,
some social infrastructures such as railway
or road, etc. should not be adjusted according
to the possibility of growth. But, most manufacturing
industries operating in the market economy
should be. Cutting the production capacity
according to the PI may cause unemployment.
But for this concern, we should examine the
case of the Textile Industry. As we have
seen in the survey of the Japanese manufacturing
industries, the production in the Textile
Industry has been stagnated. Especially,
the production in the Silk Reeling Industry
has been decreasing. Many textile companies
cut the production capacity and reduced the
production. But some textile companies had
diversified their management and their current
profit hit the highest record in their history.
For example in case of Teijin, one of the
biggest textile companies in Japan, its composition
of sales is Tetron (synthetic fiber) 52,
Nylon 9, Other Textile 3, Chemical Synthetic
Products 23, Medicine 12, Technical Plan
and Others 1 in September 1989. The company
has achieved the highest record of current
profit in its history in March 1989 (the
end of fiscal year) and is going to break
the record at the end of next fiscal year.
Other companies such as Toray Industries
and Asahi Chemical Industries are also in
the similar situation.*36
The examples of diversification can be seen
in other industries also. In case of the
Iron and Steel Industry, it is said that
"The iron is the state." Thus,
this industry is one of the most important
industries in Japan. But its status in the
world is declining due to the competition
from abroad. So some companies are also diversifying
the management into the field such as the
information, communication, electronic products,
real property, etc.*37 In the Shipbuilding Industry also, some
companies are diversifying the management.*38
It is said the life cycle of one industry
is 30 years. One industry is born, grows,
gets matured, and gets old. This period is
said to be 30 years.*39 Therefore, most industry must always adjust
its management according to the changes in
the situation.
Finally, we should examine the future perspective
of the possible contribution of the PI. Author has
specified the necessary statistics for the
PI. These statistics needs radical modification
of the existing statistical system. But if
they become available, we can work out the
more reliable PI. If some countries in the
world adopt the new statistic system for
PI, the international comparison will be
possible. The PI will be useful not only
for the developing countries but also for
the industrialized countries because there
is no country where there is no control of
the economy by the government. On of the
most remarkable international specifications
of statistics is the I-O table. The Institute
of Developing Economies in Japan sent the
experts to the ASEAN countries to instruct
the local staffs. So today, the international
I-O table, which works as an I-O table including
multiple countries, is available.*40 In the table, Indonesia, Peninsular Malaysia,
Philippines, Singapore, Thailand, Japan,
Korea, and U.S.A. are linked in one I-O table.
If the statistic system for PI is introduced
into each country like the international
I-O table, it will become a very important
data to observe the situation of world
economy.
APPENDICES
1 Source and Note of the Figures
Fig. 1-13 (From the Fig. 1 to the Fig. 13)
The graphs from A to E of 4 sectors selected
In the graph A, the relation between the
data and the line is as follows.

In the graph B, the relation between the
data and the line is as follows.

In the graph C, the relation between the
data and the line is as follows.

In the graph D, the relation between the
data and the line is as follows.
In the graph E, the relation between the
data and the line is as follows.
The unit and source of data of the Fig. 1-13
are as follows.
Fig. 1 3 CEMENT A
SOURCE
OUT and CAP: GOI, Abstract, Relevant Issues. Other data were calculated
by author.
NOTE: Unit of OUT and CAP is tonnes.
Fig. 2 3 CEMENT B
SOURCE
OUT and CAP: Same as the Fig. 1. EXPORTS:
Government of India, Directorate of Public
Relations and Publications, Directorate General
of Technical Development, Handbook of Industrial Data (New Delhi: Directorate General of Technical
Development, 1975), Relevant Issues (hereafter
cited as GOI, Handbook); K&S, Economic Guide and Investors' Handbook, 1961, p. 67; and Kothari Enterprises, Kothari's Industrial Directory of India,
1986 (Madras: Kothari Enterprises, n.d.), p.
7 (Cement) (hereafter cited as Kothari, Directory). IMPORTS: GOI, Handbook, 1966, p. 141; K&S, Economic Guide and Investors' Handbook, 1961, p. 67; and Kothari, Directory, 1986, p. 3 and 7 (Cement). ED: GOI, Guidelines, 1978/79, p. 142; GOI, Handbook, Relevant Issues; Government of India, Planning
Commission, Sixth Five Year Plan, 1980/85 (New Delhi: Planning Commission, n.d.),
p. 48 (hereafter cited as GOI, Sixth Plan); K&S, Economic & Industrial Guide, 1973/74, p. 3 and 5 (Cement); K&S, Economic Guide and Investors' Handbook, Relevant Issues; K&S, Encyclopaedia, Relevant Issues; and Kothari, Directory, 1986, p. 2 (Cement).
Fig. 3 3 CEMENT C
SOURCE
OUT and CAP: Same as the Fig. 1. O-TARGET:
Confederation of Engineering Industry, Handbook of Statistics, 1986, 19th ed. (Delhi: Confederation of Engineering
Industry, December 1986), p. 116 (hereafter
cited as CEI, Handbook); GOI, Guidelines, 1986/87, p. 113; GOI, Handbook, 1973, p. 255; K&S, Economic Guide and Investors' Handbook, 1969/70, p. 110; and Kothari, Directory, 1986, p. 3 (Cement). C-TARGET: CEI, Handbook, 1986, p. 116; GOI, Guidelines, 1986/87, p. 113; K&S, Economic & Industrial Guide,
1973/74, p. 1 (Cement); K&S, Economic Guide and Investors' Handbook, 1969/70, p. 110; K&S, Encyclopaedia, 1954, p. 2 (Cement); and Kothari, Directory, 1986, p. 3.
Fig. 4 3 CEMENT D
SOURCE
EXPORTS: GOI, Handbook, Relevant Issues. IMPORTS: GOI, Handbook, 1966, p. 141; and GOI, Sixth Plan, p. 75.
Fig. 5 3 CEMENT E
SOURCE
DO: GOI, Guidelines, 1973/74, p. 203; GOI, Handbook, 1966, p. 141; K&S, Economic & Industrial Guide, Relevant Issues; K&S, Economic Guide and Investors' Handbook, Relevant Issues; K&S, Encyclopaedia, Relevant Issues; and Kothari, Directory, 1986, p. 8. DAR: GOI, Guidelines, 1986, p. 113; K&S, Economic & Industrial Guide, Relevant Issues; K&S, Economic Guide and Investors' Handbook, Relevant Issues; K&S, Encyclopaedia, Relevant Issues; and Kothari, Directory, 1986, p. 6.
Fig. 6 6 ELECTRIC MOTORS A
SOURCE
OUT and CAP: Same as the Fig. 1. Other data
were calculated by author.
NOTE: Unit of OUT and CAP is horsepower.
Fig. 7 6 ELECTRIC MOTORS D
SOURCE
EXPORTS: GOI, Guidelines, Relevant Issues; GOI, Handbook, 1975, p. 87; K&S, Economic & Industrial Guide, 1978/79, p. 28 (Engineering); K&S, Economic Guide and Investors' Handbook, 1961, p. 117; and K&S, Encyclopaedia, 1956/57, p. 542. IMPORTS: GOI, Guidelines, 1979/80, p. 14; GOI, Handbook, 1975, p. 87; K&S, Economic Guide and Investors' Handbook, Relevant Issues; and K&S, Encyclopaedia, 1956/57, p. 541.
Fig. 8 6 ELECTRIC MOTORS E
SOURCE
DO: GOI, Guidelines, Relevant Issues; GOI, Handbook, 1966, p. 41; K&S, Economic Guide and Investors' Handbook, Relevant Issues; and K&S, Encyclopaedia, Relevant Issues. DAR: GOI, Guidelines, Relevant Issues; GOI, Handbook, Relevant Issues; K&S, Economic Guide and Investors' Handbook, 1961, p. 116; and K&S, Encyclopaedia, 1960, pp. 170-1.
Fig. 9 7 CERAMIC INSULATORS A
SOURCE
OUT and CAP: Same as the Fig. 1. Other data
were calculated by author.
NOTE: Unit of OUT and CAP is numbers from
1951 to 1956 and thereafter tonnes.
Fig. 10 7 CERAMIC INSULATORS E
SOURCE
DO: Government of India, Ministry of Commerce
and Industry, Development Commissioner (Small
Scale Industries), Teiatsu You Zetsuen Touki, (Toubu Chihou) [The title in English is not known], Hon'yaku
Shiryou no. 27, Indo Chushoukougyou Shirizu,
no. 27, trans. Osaka Ajia Chushoukigyou Kaihatsu
Senta (n. p. : Osaka Ajia Chushoukigyou Kaihatsu
Senta, 1965), Relevant Pages (hereafter cited
as GOI, Zetsuen Touki); and K&S, Encyclopaedia, Relevant Issues. DAR: GOI, Zetsuen Touki, Relevant Pages; and K&S, Encyclopaedia, Relevant Issues.
Fig. 11 8 LEAD A
SOURCE
OUT and CAP: Same as the Fig. 1. Other data
were calculated by author.
NOTE: Unit of OUT and CAP is tonnes.
Fig. 12 8 LEAD B
SOURCE
OUT and CAP: Same as the Fig. 1. EXPORTS:
GOI, Handbook, 1975, p. 59. IMPORTS: GOI, Guidelines, Relevant Issues; GOI, Handbook, Relevant Issues; K&S, Economic & Industrial Guide, 1978/79, p. 28 (Minerals & Metals); K&S,
Economic Guide and Investors' Handbook, 1961, p. 245 (Mining & Chemicals). ED: GOI,
Guidelines, Relevant Issues; GOI, Handbook, 1973, p. 149; GOI, Sixth Plan, p. 49; and K&S, Economic Guide and Investors' Handbook, Relevant Issues.
Fig. 13 8 LEAD E
SOURCE
DC: GOI, Handbook, 1973, p. 149. DO: GOI, Guidelines, Relevant Issues; GOI, Handbook, 1966, p. 59; K&S, Economic & Industrial Guide, Relevant Issues; K&S, Economic Guide and Investors' Handbook, Relevant Issues; and K&S, Encyclopaedia, Relevant Issues. DAR: GOI, Guidelines, Relevant Issues; K&S, Economic Guide and Investors' Handbook, Relevant Issues; K&S, Encyclopaedia, Relevant Issues; and Koti, pp. 74-5.
Fig. 14-15 Profitability of 11 sectors selected
SOURCE: GOI, ASI, Relevant Issues.
Fig. 16-17 Relative value of profitability of the cement
and the electric motors among 11 sectors
SOURCE: same as the Fig. 14-15.
Fig. 18-21 The out put, the production capacity, and the
capacity utilization of 4 sectors in Japan
SOURCE: MITI, Nenpou, 1987, pp. 70-8; and ----------, Souran, Relevant Issues.
NOTE: The data are shown by the index with
the base year 1980.
Fig. 22-23 The exports and the imports of the Chemical
Fertilizer and the Silk Reeling
SOURCE: Nihon Kanzei Kyoukai, ed., Gaikoku Boueki Gaikyou (Tokyo: Nihon Kanzei Kyoukai, Relevant Issues).
Fig. 24-25 The PI of 9 sectors in India
NOTE: The numbers beneath the graph are the
code number of 9 sectors.
Fig. 26-27 The rank of PI of 9 sectors in India
NOTE: The numbers beneath the graph are the
code number of 9 sectors.
2 Figures
Fig. 1

Fig. 2
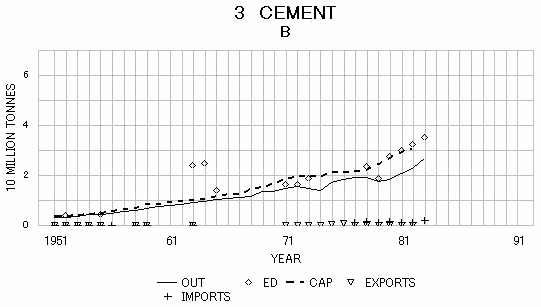
Fig. 3

Fig. 4
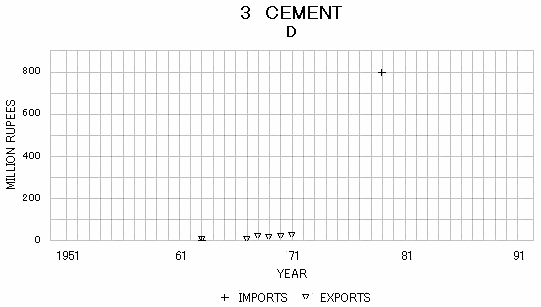
Fig. 5

Fig. 6

Fig. 7
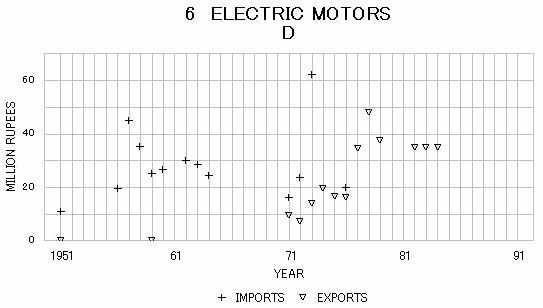
Fig. 8

Fig. 9
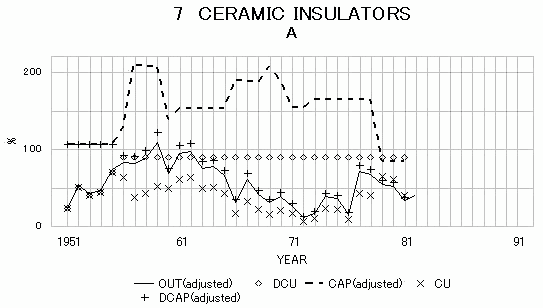
Fig. 10
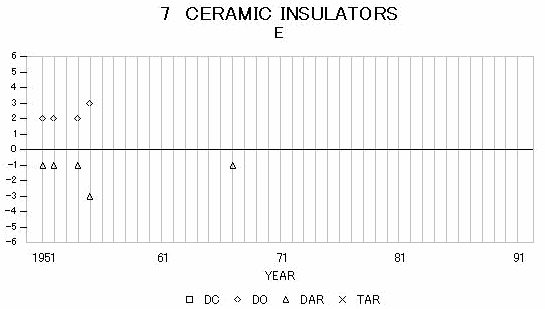
Fig. 11
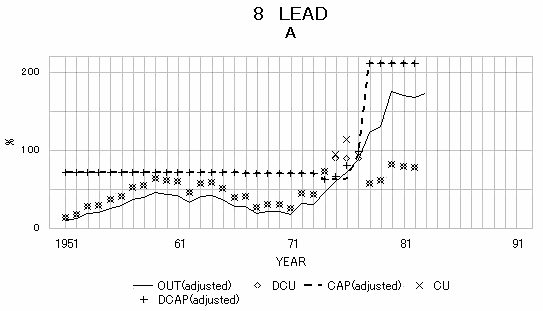
Fig. 12

Fig. 13
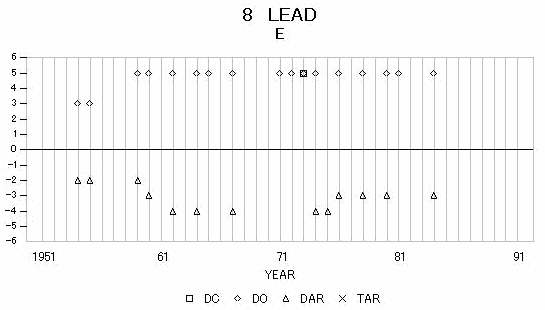
Fig. 14

Fig. 15
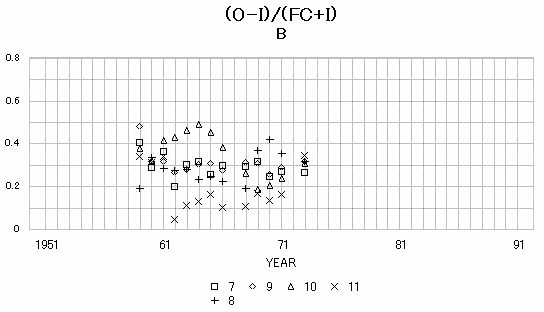
Fig. 16

Fig. 17

Fig. 18
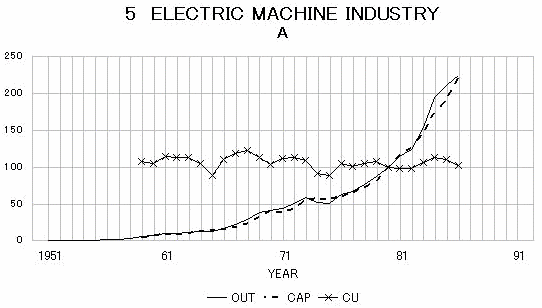
Fig. 19

Fig. 20

Fig. 21

Fig. 22
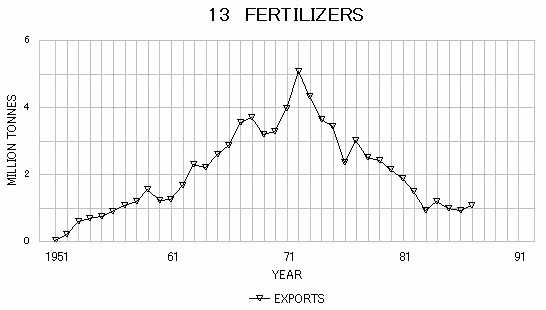
Fig. 23
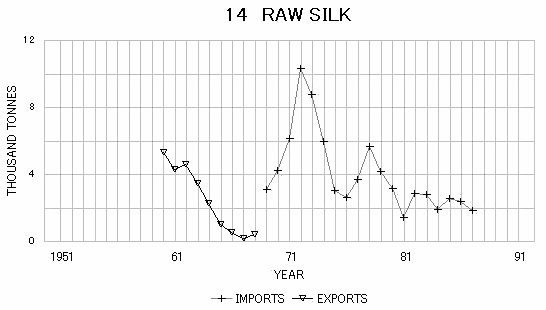
Fig. 24
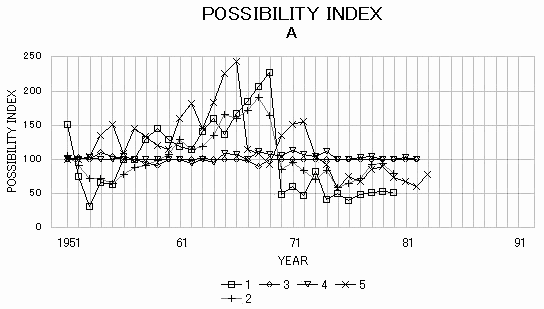
Fig. 25
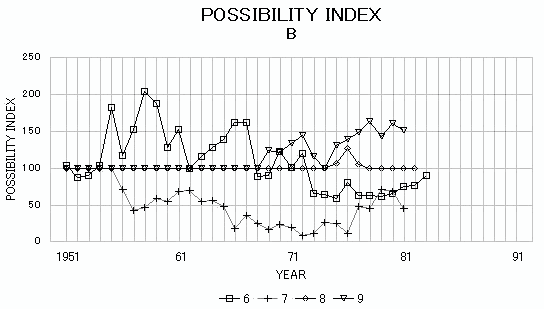
Fig. 26
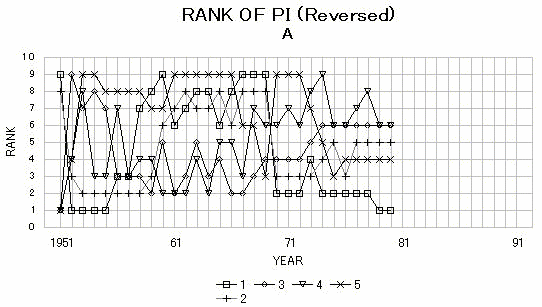
Fig. 27
3 TABLES
TABLE 1
INVESTMENT THEORIES, FACTORS TAKEN INTO ACCOUNT,
AND DETERMINANT FACTORS OF INVESTMENT
| Name of Theory |
Factors Taken into Account |
Determinant Factor of Investment |
| Capital-Turnover Criterion |
rate of capital-turnover (output-capital
ratio) |
rate of capital- turnover |
Social Marginal Productivity (SMP) Criterion
|
SMP (average annual increment in national
income from the marginal unit of investment),
increment in capital (investment),
output,
external economies,
balance of payment,
labor cost,
overhead cost,
cost of materials |
SMP
|
Reinvestment Criterion
|
saving from investment,
productivity of labor,
population |
amount of saving per unit of additional capital
|
Time Series Criterion
|
period of recovery (gestation period of project),
rate of capital-turnover,
SMP,
Reinvestment Criterion |
period of recovery
|
Ratio of Labor to Investment Criterion
|
employment,
investment |
employment per unit of additional capital
|
| Balanced Growth and Unbalanced Growth |
balance between sectors or linkage effect
|
selection between the investment into leading
sectors and the investment into group of
projects |
| Cost-Benefit Analysis |
cost-benefit ratio of investment |
cost-benefit ratio of investment |
Theories on External Economies and Economies
of Scale
|
external economies,
economies of scale,
import price,
production cost |
make-buy decision depending on import price
and production cost |
Marginal Efficiency of Capital
|
interest rate,
return from investment,
marginal efficiency of capital |
interest rate
|
Acceleration Principle
|
capital-output ratio (accelerator),
capital stock,
output,
national income,
time lag of investment,
capacity utilization |
change in output
|
Neoclassical Theory
|
output,
capital stock,
labor,
interest rate,
revenue before tax,
price of capital goods,
price of output,
rate of investment,
wage rate,
user cost of capital ,
taxes,
replacement of capital stock,
delivery lag of investment,
desired capital stock,
net worth |
user cost of capital
|
Tobin's q Theory
|
market value of firm,
replacement cost of capital,
adjustment cost,
capital stock,
labor,
output,
price of output,
wage rate,
capital cost |
q (ratio between market value of firm and
replacement cost of capital)
|
Possibility Index (PI)
|
output,
production capacity,
capacity utilization,
profitability,
availability of raw materials,
imports and exports,
ratio between the demand and supply in the
market,
estimated demand |
PI (ratio between the desired capacity and
the actual capacity)
|
SOURCE: Summarized from section II.
TABLE 2
CAPACITY UTILIZATION OF SELECTED 4 SECTORS
IN INDIA
| Year |
Sector
3
(%) |
Sector
6
(%) |
Sector
7
(%) |
Sector
8
(%) |
1951
52
53
54
55
56
57
58
59
60
61
62
63
64
65
66
67
68
69
70
71
72
73
74
75
76
77
78
79
80
81
82
83
|
89.8
93.2
90.2
98.9
94.6
86.4
85.7
86.0
82.0
90.0
87.0
88.3
91.0
90.0
90.5
88.5
88.5
80.9
87.6
82.3
80.7
79.8
75.9
72.1
80.3
87.0
88.1
87.0
72.2
69.1
72.0
73.6
|
93.5
79.0
81.0
94.0
164.7
105.6
137.9
183.5
169.4
115.6
137.7
86.8
104.6
115.7
125.7
146.4
146.4
80.5
80.8
111.1
90.7
107.7
59.6
57.7
53.1
72.5
57.4
57.1
55.1
59.3
68.2
69.0
81.8
|
23.6
50.7
40.5
44.1
70.4
64.6
38.8
42.7
42.6
49.4
61.5
63.5
49.6
50.6
43.0
17.0
32.8
22.6
15.6
20.9
17.6
7.7
10.7
23.8
22.6
9.9
43.8
40.8
64.8
61.9
40.7
|
14.3
18.9
28.2
29.8
37.2
41.6
52.9
55.6
64.9
62.0
60.1
46.7
58.0
59.4
52.4
40.7
41.2
27.5
31.1
31.0
25.7
45.7
43.9
73.8
95.5
14.5
94.5
58.0
62.1
82.9
80.3
79.2
|
NOTE: The number of sector is the number
of 9 sectors selected.
TABLE 3
THE PI OF SELECTED 9 SECTORS IN INDIA
| Year |
Sector 1 |
Sector 2 |
Sector 3 |
Sector 4 |
Sector 5 |
Sector 6 |
Sector 7 |
Sector 8 |
Sector 9 |
1951
52
53
54
55
56
57
58
59
60
61
62
63
64
65
66
67
68
69
70
71
72
73
74
75
76
77
78
79
80
81
82
83
|
151.8
74.6
31.4
66.2
63.9
100.0
100.0
129.2
145.2
129.0
119.7
114.8
141.3
160.3
137.2
166.9
184.8
206.5
227.5
48.8
60.3
47.3
83.1
41.5
50.3
40.7
48.9
52.5
53.6
51.4
|
105.5
91.1
71.8
72.1
65.3
78.6
88.1
91.5
98.8
06.8
29.9
14.8
19.0
34.6
65.6
60.8
72.4
90.4
64.3
85.3
95.4
83.9
71.6
83.6
59.5
65.3
72.9
92.4
94.7
79.5
|
100.0
103.5
100.3
109.9
105.1
100.0
100.0
95.5
91.1
100.1
100.0
100.0
101.1
100.0
100.5
100.0
98.3
89.9
100.0
100.0
100.0
100.0
100.0
100.0
100.0
100.0
100.0
100.0
100.0
100.0
100.0
100.0
|
100.0
100.0
103.3
100.0
100.0
104.0
100.0
100.0
100.0
100.0
100.0
94.8
100.2
95.1
108.5
107.1
100.0
111.9
107.8
105.6
113.0
108.0
104.5
111.6
100.0
100.0
102.9
104.5
100.0
100.0
103.3
100.0
|
100.0
100.0
104.3
135.2
151.6
109.3
145.0
134.1
120.9
114.5
160.4
182.5
146.1
184.1
226.0
244.0
115.2
108.5
93.4
134.9
151.4
155.6
104.4
92.5
58.3
75.6
67.3
85.3
89.8
73.9
67.5
60.5
77.7
|
103.8
87.8
90.0
104.4
183.0
117.3
153.3
203.9
188.2
128.5
153.0
100.0
116.2
128.5
139.6
162.7
162.7
89.4
89.8
123.4
100.8
119.7
66.2
64.1
59.0
80.6
63.8
63.5
61.3
65.9
75.8
76.7
90.9
|
100.0
100.0
100.0
100.0
100.0
71.8
43.1
47.5
58.5
54.8
68.3
70.6
55.1
56.2
47.8
18.9
36.5
25.1
17.3
23.2
19.5
8.6
11.9
26.4
25.1
11.0
48.7
45.4
72.0
68.8
45.2
|
100.0
100.0
100.0
100.0
100.0
100.0
100.0
100.0
100.0
100.0
100.0
100.0
100.0
100.0
100.0
100.0
100.0
100.0
100.0
100.0
100.0
100.0
100.0
100.0
106.1
127.2
105.0
100.0
100.0
100.0
100.0
100.0
|
100.0
100.0
100.0
100.0
100.0
100.0
100.0
100.0
100.0
100.0
100.0
100.0
100.0
100.0
100.0
100.0
100.0
100.0
124.1
121.4
134.0
144.7
116.4
100.0
131.5
138.7
148.8
163.6
143.8
161.0
151.4
|
NOTE: The number of sector is the code number
of 9 sectors selected.
NOTES
*1 J. J. Polak, "Balance of Payments Problems
of Countries Reconstructing with the Help
of Foreign Loans," Quarterly Journal of Economics 57 (February 1943): 208-40; and Norman S.
Buchanan, International Investment and Domestic Welfare (New York: Henry Colt, 1945), quoted in
Subrata Ghatak, An Introduction to Development Economics, 2nd ed. (London: Allen & Unwin, 1986),
p. 85.
*2 Alfred E. Kahn, "Investment Criteria
in Development Programs," Quarterly Journal of Economics 65 (February 1951): 38-61.
*3 Hollis B. Chenery, "The Application
of Investment Criteria," Quarterly Journal of Economics 67 (February 1953): 76-96.
*4 Walter Galenson and Harvey Leibenstein,
"Investment Criteria, Productivity,
and Economic Development," Quarterly Journal of Economics 69 (August 1955): 343-70.
*5 Amartya K. Sen, "Some Notes on the
Choice of Capital-Intensity in Development
Planning," Quarterly Journal of Economics 71 (November 1957): 561-84.
*6 Krishna K. Singh, Investment Project in A Planned Economy (Delhi: Amar Prakashan, 1985), pp. 22-5.
*7 Ragnar Nurkse, Problems of Capital Formation in Under Developed
Countries (Oxford: Basil Blackwell Publisher Ltd.,
1953; reprint ed., Delhi: Oxford University
Press, 1973).
*8 Paul N. Rosenstein-Rodan, "Problems
of Industrialization of Eastern and South-Eastern
Europe," Economic Journal 53 (June- September 1943): 202-11; and Nurkse.
*9 Albert O. Hirschman, "The Strategy
of Economic Development," Precis of
a lecture delivered at the Institute on ICA
Development Programming; reprinted in A.
N. Agarwala and S. P. Singh, ed., Accelerating Investment in Developing Economies (London: Oxford University Press, 1969),
pp. 3-11; Charles P. Kindleberger, The Terms of Trade: A European Case Study (New York: Technology Press/Wiley, 1956),
quoted in Ghatak, p. 98; and Paul Streeten,
"Unbalanced Growth," Oxford Economic Papers 11 (June 1959): 167-90.
*10 Tibor Scitovsky, "Two Concepts of External
Economies," Journal of Political Economy 62 (April 1954): 143-51.
*11 Hollis. B. Chenery, "The Interdependence
of Investment Decisions: Essays in Honor
of Bernard Francis Haley," in The Allocation of Economic Resources, ed. Moses Abramovitz et al (Stanford: Stanford
University Press, 1959), pp. 82-120.
*12 Larry E. Westphal, "Planning with Economies
of Scale," in Economy Wide Models and Development Planning, ed. Charles R. Blitzer, Peter B. Clark,
and Lance Taylor (Oxford: Oxford University
Press, 1975), pp. 257-306.
*13 Hollis B. Chenery and Larry E. Westphal,
"Economies of Scale and Investment over
Time," in Structural Change and Development Policy, A World Bank Research Publication, ed.
Hollis B. Chenery (n.p.: Oxford University
Press, 1979), pp. 217- 67.
*14 Larry E. Westphal and Jacques Cremer, "'The
Interdependence of Investment Decisions'
Revisited," in Economic Structure and Performance, ed. Moshe Syrquin, Lance Taylor, and Larry
E. Westphal (Orlando: Academic Press, Inc.,
1984), pp. 543-72.
*15 John M. Keynes, The General Theory of Employment, Interest
and Money (London: Macmillan & Co., Ltd., 1936;
reprint 4th ed., Tokyo: Maruzen Co., Ltd.,
1973), p. 135.
*16 Hollis B. Chenery, "Overcapacity and
the Acceleration Principle," Econometrica 20 (January 1952): 1-28.
*17 Dale W. Jorgenson, "Capital Theory
and Investment Behavior," American Economic Review 53 (May 1963): 247-59.
*18 James Tobin, "A General Equilibrium
Approach to Monetary Theory," Journal of Money, Credit and Banking 1 (February 1969): 15-29.
*19 Government of India, Ministry of Planning,
Department of Statistics, Central Statistical
Organization, Statistical Abstract (New Delhi: Central Statistical Organization,
Relevant Issues), (hereafter cited as GOI,
Abstract).
*20 Raghunath K. Koti, Utilization of Industrial Capacity in India,
1967-68, Gokhale Institute Mimeograph Series No.
9 (Poona: Gokhale Institute of Politics and
Economics, n.d.), pp. 91-9.
*21 Government of India, Ministry of Planning,
Department of Statistics, Central Statistical
Organization, Annual Survey of Industries: Census Sector (Calcutta: Central Statistical Organization,
Relevant Issues) (hereafter cited as GOI,
ASI).
*22 The number in the parenthesis is the number
of 9 sectors selected.
*23 Kothari & Sons, Kothari's Economic and Industrial Guide of
India, 1978-79 (Madras: Kothari & Sons, n.d.), p. 4
(Cement) (hereafter cited as K&S, Economic & Industrial Guide).
*24 For example, K&S, Economic & Industrial Guide, 1973-1974, p. 2-3 (Cement).
*25 K&S, Economic & Industrial Guide, 1973-74, p. 2 (Cement); and K&S, Economic & Industrial Guide, 1976, p. 1 (Cement).
*26 K&S, Economic & Industrial Guide, 1976, p. 4 (Cement).
*27 Kazuo Osugi ed., Indo: Keizai to Toushikankyou (Tokyo: Institute of Developing Economies,
1968), pp. 306-7.
*28 Kothari & Sons, Kothari's Economic Guide and Investors' Handbook
of India, 1969-1970, (Madras: Kothari & Sons, n.d.), p.
111 (hereafter cited as K&S, Economic Guide & Investors' Handbook).
*29 Government of India, Ministry of Industry,
Department of Industrial Development, Indian
Investment Centre, Guidelines for Industries, 1973-74 (New Delhi: Indian Investment Centre, 1973),
p. 202 (hereafter cited as GOI, Guidelines).
*30 Kothari & Sons, Investors' Encyclopaedia, 1955-56 (Madras: Kothari & Sons, n.d.), p. 16
(Mining & Chemicals) (hereafter cited
as K&S, Encyclopaedia).
*31 Koti, summarized from p. 29 and 45.
*32 Tsushousangyou Daijin Kanbou Chousa Toukeibu, ed. Koukougyou ShisuSouran (Tokyo: Okurashou Insatsukyoku, Relevant
Issues) (hereafter cited as MITI (Ministry
of International Trade and Industry), Souran); and ----------, Koukougyou Shisu Nenpou, 1981 (Tokyo: Okurashou Insatsukyoku, 1981), pp.
64-74, and pp. 164-7 (hereafter cited as
MITI, Nenpou).
*33 Okurashou, Zaisei Kin'yu Toukei Geppou, Houjin Kigyou Toukei Nenpou Tokushu, (Tokyo:
Okurashou Insatsukyoku, Relevant Issues)
(hereafter cited as Okurashou, Zaisei Geppou); Okurashou Rizaikyoku Keizaika, Houjin Kigyou Toukei Nenpou, 1960 (Tokyo: Okurashou Insatsukyoku, 1960), Relevant
Pages (hereafter cited as Okurashou, Kigyou Toukei).
*34 Nihon Keizai Shinbunsha, ed., Zeminaru Nihon Keizai Nyumon (Tokyo: Nihon Keizai Shinbunsha, 1987),
p. 31.
*35 Hisao Kanamori, Kouza Nihon Keizai - Jou: Keizai Shakai no
Shikumi (Tokyo: Chuoukeizaisha, 1982), p. 11.
*36 Touyou Keizai, Kaisha Shikihou, First Series in 1990 (Tokyo: Touyou Keizai,
December 1989), pp. 179-81.
*37 Touyou Keizai, pp. 350-2.
*38 Touyou Keizai, pp. 612-4.
*39 Nihon Keizai Shinbunsha, p. 29.
*40 Institute of Developing Economies (I.D.E),
International Input-Output Table for ASEAN
Countries, 1975, I.D.E. Statistical Series, no. 39 (Tokyo:
Institute of Developing Economies, 1982).
SELECTED BIBLIOGRAPHY
1 Bibliography in English
Agarwala, A. N., and Singh S. P., ed. Accelerating Investment in Developing Economies. London: Oxford University Press, 1969.
Buchanan, Norman S. International Investment and Domestic Welfare. New York: Henry Colt, 1945. Quoted in Subrata
Ghatak, An Introduction to Development Economics, 2nd ed., p. 85. London: Allen & Unwin,
1986.
Chenery, Hollis B. "Overcapacity and
the Acceleration Principle." Econometrica 20 (January 1952): 1-28.
----------. "The Application of Investment
Criteria." Quarterly Journal of Economics 67 (February 1953): 76-96.
----------. "The Interdependence of
Investment Decisions." In The Allocation of Economic Resources: Essays
in Honor of Bernard Francis Haley, pp. 82-120. Edited by Moses Abramovitz
et al. Stanford: Stanford University Press,
1959.
----------, and Westphal, Larry E. "Economies
of Scale and Investment over Time."
In Structural Change and Development Policy, A World Bank Research Publication, pp.
217-67. Edited by Hollis B. Chenery. n.p.:
Oxford University Press, 1979.
Confederation of Engineering Industry. Handbook of Statistics, 1986. 19th ed. Delhi: Confederation of Engineering
Industry, December 1986.
Galenson, Walter, and Leibenstein, Harvey.
"Investment Criteria, Productivity,
and Economic Development." Quarterly Journal of Economics 69 (August 1955): 343-70.
Ghatak, Subrata. An Introduction to Development Economics. 2nd ed. London: Allen & Unwin, 1986.
Hirschman, Albert O. "The Strategy of
Economic Development." Precis of a lecture
delivered at the Institute on ICA Development
Programming; reprinted in Agarwala and S.
P. Singh, pp. 3-11.
India, Government of, Directorate of Public
Relations and Publications, Directorate General
of Technical Development. Handbook of Industrial Data. New Delhi: Directorate General of Technical
Development, Relevant Issues.
----------, Ministry of Industry, Department
of Industrial Development, Indian Investment
Centre. Guidelines for Industries. New Delhi: Indian Investment Centre, Relevant
Issues.
----------, Ministry of Planning, Department
of Statistics, Central Statistical Organization.
Annual Survey of Industries: Census Sector. Calcutta: Central Statistical Organization,
Relevant Issues.
----------, ----------, ----------. Statistical Abstract. New Delhi: Central Statistical Organization,
Relevant Issues.
----------, Planning Commission. Sixth Five Year Plan, 1980/85. New Delhi: Planning Commission, n.d.
Institute of Developing Economies (I.D.E).
International Input-Output Table for ASEAN
Countries, 1975. I.D.E. Statistical Series, no. 39. Tokyo:
Institute of Developing Economies, 1982.
Jorgenson, Dale W. "Capital Theory and
Investment Behavior," American Economic Review 53 (May 1963): 247-59.
Kahn, Alfred E. "Investment Criteria
in Development Programs." Quarterly Journal of Economics 65 (February 1951): 38-61.
Keynes, John M. The General Theory of Employment, Interest
and Money. London: Macmillan & Co., Ltd., 1936;
reprint 4th ed., Tokyo: Maruzen Co., Ltd.,
1973.
Kindleberger, Charles P. The Terms of Trade: A European Case Study. New York: Technology Press/Wiley, 1956.
Quoted in Ghatak, p. 98.
Kothari & Sons. Investors' Encyclopaedia. Madras: Kothari & Sons, Relevant Issues.
----------. Kothari's Economic and Industrial Guide of
India. Madras: Kothari & Sons, Relevant Issues.
----------. Kothari's Economic Guide and Investors' Handbook
of India. Madras: Kothari & Sons, Relevant Issues.
Kothari Enterprises. Kothari's Industrial Directory of India,
1986. Madras: Kothari Enterprises, n.d.
Koti, Raghunath K. Utilization of Industrial Capacity in India,
1967-68. Gokhale Institute Mimeograph Series, No.
9. Poona: Gokhale Institute of Politics and
Economics, n.d.
Nurkse, Ragnar. Problems of Capital Formation in Underdeveloped
Countries. Oxford: Basil Blackwell Publisher Ltd.,
1953; reprint ed., Delhi: Oxford University
Press, 1973.
Polak, J. J. "Balance of Payment Problems
of Countries Reconstructing with the Help
of Foreign Loans." Quarterly Journal of Economics 57 (February 1943): 208-40.
Rosenstein-Rodan, Paul N. "Problems
of Industrialization in Eastern and South-Eastern
Europe." Economic Journal 53 (June-September 1943): 202-11.
Scitovsky, Tibor. "Two Concepts of External
Economies." Journal of Political Economy 62 (April 1954): 143-51.
Sen, Amartya K. "Some Note on the Choice
of Capital-Intensity in Development Planning."
Quarterly Journal of Economics 71 (November 1957): 561-84.
Singh, Krishna K. Investment Project in A Planned Economy. Delhi: Amar Prakashan, 1985.
Streeten, Paul. "Unbalanced Growth."
Oxford Economic Papers 11 (June 1959): 167-90.
Tobin, James. "A General Equilibrium
Approach to Monetary Theory." Journal of Money, Credit and Banking 1 (February 1969): 15-29.
Westphal, Larry E. "Planning with Economies
of Scale," In Economy Wide Models and Development Planning, pp. 257-306. Edited by Charles R. Blitzer,
Peter B. Clark, and Lance Taylor. Oxford:
Oxford University Press, 1975.
----------, and Cremer, Jacques. "'The
Interdependence of Investment Decisions'
Revisited," In Economic Structure and Performance, pp. 543-72. Edited by Moshe Syrquin, Lance
Taylor, and Larry E. Westphal. Orlando: Academic
Press, Inc., 1984.
2 Bibliography in Japanese
India, Government of, Ministry of Commerce
and Industry, Development Commissioner (Small
Scale Industries). Teiatsu You Zetsuen Touki, (Toubu Chihou) [The title in English is not known.]. Hon'yaku
Shiryou no. 27. Indo Chushoukougyou Shirizu,
no. 27. Translated by Osaka Ajia Chushoukigyou
Kaihatsu Senta. n.p.: Osaka Ajia Chu-shoukigyou
Kaihatsu Senta, 1965.
Kanamori, Hisao. Kouza Nihon Keizai - Ge: Keizai Shakai no
Shikumi. Tokyo: Chuoukeizaisha, 1982.
----------. Kouza Nihon Keizai - Jou: Keizai Shakai no
Shikumi. Tokyo: Chuoukeizaisha, 1982.
Nihon Kanzei Kyoukai, ed. Gaikoku Boueki Gaikyou. Tokyo: Nihon Kanzei Kyoukai, Relevant Issues.
Nihon Keizai Shinbunsha, ed. Zeminaru Nihon Keizai Nyumon. Tokyo: Nihon Keizai Shinbunsha, 1987.
Okurashou. Zaisei Kin'yu Toukei Geppou. Houjin Kigyou Toukei Nenpou Tokushu. Tokyo:
Okurashou Insatsukyoku, Relevant Issues.
----------, Rizaikyoku Keizaika. Houjin Kigyou Toukei Nenpou, 1960. Tokyo: Okurashou Insatsukyoku, 1960.
Touyou Keizai. Kaisha Shikihou. First Series in 1990. Tokyo: Touyou Keizai,
December 1989.
Tsushousangyou Daijin Kanbou Chousa Toukeibu,
ed. Koukougyou Shisu Nenpou. Tokyo: Okurashou Insatsukyoku, Relevant
Issues.
----------. Koukougyou Shisu Souran. Tokyo: Okurashou Insatsukyoku, Relevant
Issues.
|